View Distance Between Foci Of Ellipse Pics. If the major axis and minor axis are the same length, the however if you have an ellipse with known major and minor axis lengths, you can find the location of the foci using the formula below. If you take any point on the ellipse, the sum of the distances to those 2 fixed points ( blue tacks ) is constant.

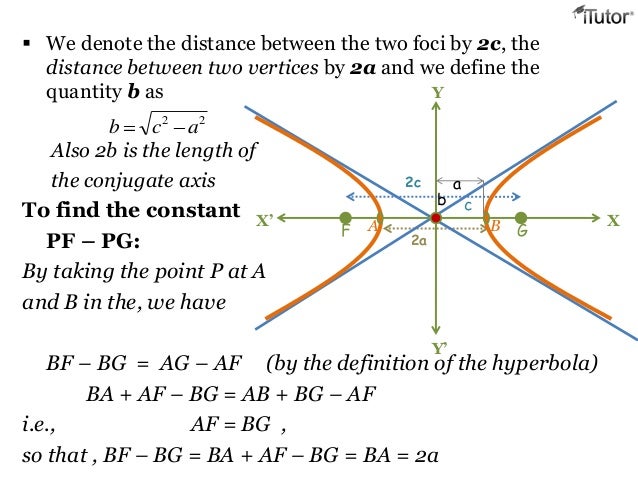
Equation of Hyperbola from image.slidesharecdn.com

The foci are $b,d$, $ef$ bisects $bd$ and the point of. If the eccentricity is zero the foci match with the center point and become a circle. The foci always lie on the major (longest) axis, spaced equally each side of the center.

Then the length of the semi major axis is.

Then the length of the semi major axis is. A circle is a special case of an ellipse with no eccentricity, and both foci are on top of each other: Given an ellipse $e$ (with the foci $f_1$ and $f_2$ and the center $c$), and a point $p$, which is the maximum distance that $p$ can be to all these 3 the other is at an $x$ coordinate halfway between the center and focus. This section focuses on the four variations of the standard form of the equation for the ellipse.